The Resolving Power of Telescopes
Resolving power of a telescope refers to the ability of a telescope to detect the small details. This article will explain this term so that you can grasp it easily and provide a lot of photographs and drawings. Firstly, let’s look at a double star.
What is resolving power? It is the ability of a telescope to see really small details. In this article I give you a really good understanding of what the resolving power of a telescope is and I show you lots of pictures and drawings. A good explanation of this would be to look at a double star.

Fig: View of a double star through a small telescope
If you look through a small telescope and point it to a double star, you will see this fuzzy view. Notice that the stars blend together here. This can be an example of resolving power of telescope. The ability of detecting small details or the. The resolving power of a small telescope is less. That is why the sharpness or resolution of the picture we get from it is less. What is the solution here? You can suggest to use higher magnification and get it sharper.

Fig 2: View of the same double star with a higher magnification through the same small telescope.
The picture shows you how the same double star looks if you use higher magnification. From the picture, it is clear that higher magnification gives you a bigger image, but not a better resolution. This is why you get the same fuzzy blend look of the double star.
So magnification is not all you need to make the view of the telescope better. While you are using the highest resolution of your telescope, you have to understand that magnifying any view will not make the view better or more clear.
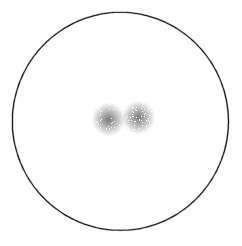
Fig 3: View of the same double star through a larger telescope.
If you look at the same double star with a larger telescope having larger primary mirror or lens, even if the magnification is like the figure 1, you will be able to see the two separate binary stars at the same distance in the view. It is the higher resolution that made the difference between the views of the same object here. It gave a sharper and more detailed and clear view.
Higher resolution means it works on more pixels per inch of the image, which allows more detail per inch of the view. If you think about television, you will understand this better. Latest high definition television will give you a clearer picture than the older television with poor resolution.
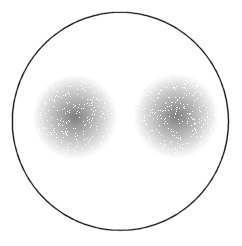
Fig 4: View of the same double star through the same larger telescope but with higher magnification same as figure 2
This picture shows you that even if you magnify the view of the larger telescope, you will get the same detail as figure 3, only difference here is the picture is bigger. This view is not clear enough to understand the double star system properly. This is why astronomers always feel the need for larger and larger telescopes. The larger the telescope, the better the resolution and the better the view you will get.
Technical aspects of Resolving power
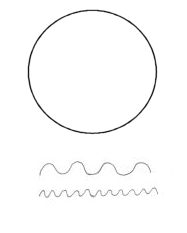
Fig 5: Objective Lense (on the top), light wave with larger wavelength (the upper wave), light wave with smaller wavelength (at the bottom)
It is known that light has a spectrum of different colors. And different color lights are different in speed, frequency and wavelength. Wavelengths play an important role in resolution.If the light that falls on a telescope lens is of larger wavelength, the telescope can capture more light which causes the higher resolution i.e you will get a clearer view. If the wavelength of the lightwave that falls on the objective lense is less, the telescope can sample less light and in turn gives you a fuzzy image for less resolution. When the wavelength is more, the telescope can gather more information and create a more accurate image.
In short, the higher the wavelength of the light, the better the resolution of the image.
Other factors affecting resolution
- The quality of the telescope – It is the quality of optics on which the quality of the telescope depends. A better optics will be able to gather more information (light) and in turn produce a better picture with more detail.
- The Atmosphere – Atmosphere is a very important factor while observing through a telescope. Whatever telescope you use, if the weather is turbulent, it will cause distortion of light and create a fuzzy image.
- The Formula: The resolution of a telescope is measured as the minimum resolvable angle in arc seconds. The formula is
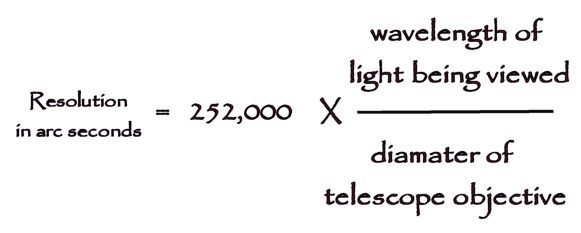
Fig 6: Formula of the resolution of telescope.
In this formula you will get two variables and a constant, where the value of the constant is 252,000 and the two variables are the diameter of the telescope (in centimeters) and the wavelength of the light (in centimeters).
The formula describes that the resolution of a telescope is 252,000 times the ratio of the wavelength of the light and the diameter of the telescope. This relation determines that the higher the diameter of the scope, the smaller the arc of the angle that can be resolved. And smaller the arc, the better the resolution.
How to Calculate the Resolving Power of a Telescope?
To calculate resolving power of a telescope divide a constant, determined by Dawe’s limit, by the aperture. This calculation is often referred to as Dawes’ formula, which divides 4.56 by the aperture diameter for imperial calculations. The Dawes’ limit is the highest level of detail or the smallest separation that a telescope is able to resolve, expressed in terms of angular resolution. The formula for calculating resolving power in inches is demonstrated below.
Resolving Power (in arcseconds) = 4.56 / Aperture (in inches)
If one wishes to calculate in metric units, the formula will be adjusted. Instead of using 4.56, observers will divide 116 by the aperture in millimeters.
Resolving Power (in arcseconds) = 116 / Aperture (in millimeters)
For example, if one has a telescope with an aperture of 100mm, they can use the second equation to calculate the resolving power. Plugging the aperture size into the equation shows a resolving power of 1.16 arcseconds.
Resolving Power = 116 / 100 = 1.16 arcseconds
This means that this specific telescope has the ability to discern details or separate celestial objects as close as 1.16 arcseconds apart from each other.
While Dawes’ limit offers a simple and generally accepted rule for estimating the resolving power, it is not a definitive measure. Other factors such as the quality of the telescope’s optics and the viewing conditions also influence the actual resolving power. The quality of optics encompasses multiple concepts, including alignment, coating and imperfections. While it’s a useful formula, it’s not an absolute measure of a telescope’s performance.
Understanding the resolving power of a telescope is crucial as it provides a standard of comparison between different telescopes, helping astronomers and enthusiasts alike make informed decisions about which instrument would best suit their observational needs. Knowing the resolving power will also guide the observer about the level of detail they can expect to discern when viewing various celestial objects.
The resolving power is particularly vital when observing celestial bodies with fine details or closely spaced objects, as a higher resolving power allows telescopes to distinguish between these with more clarity.
It’s also important to consider the relationship between resolving power and magnification. While magnification refers to the apparent enlargement of an observed object, it does not inherently improve the detail of the image. Without sufficient resolving power, increasing the magnification will merely result in a larger but blurrier image. Thus, resolving power and magnification are intertwined aspects that collectively determine the effectiveness of a telescope’s observation capabilities. 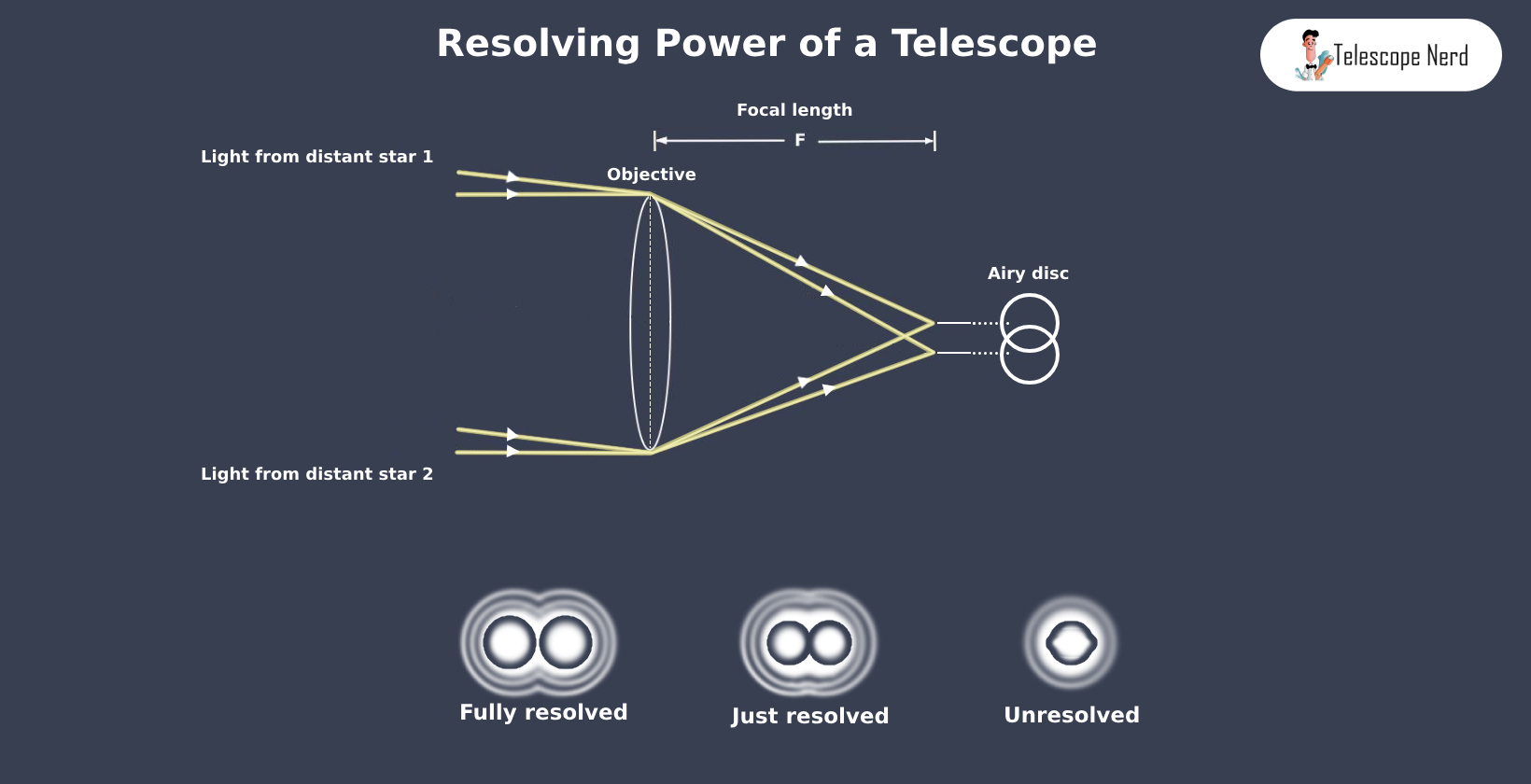
Is a Higher Resolution Telescope Better For Observation?
Yes, telescopes with higher resolution provide superior observation capabilities, primarily due to their ability to produce more detailed and intricate images. Understanding resolving power enhances the observer’s capacity to discern and study minute features of celestial bodies that would otherwise remain obscure with lower-resolution telescopes.
For example, higher resolution reveals the surface textures of planets, the structures within galaxies, and the shapes and compositions of various astronomical phenomena. It also enables the observation of objects that are closer together in the sky, such as binary stars, which will appear as a single point in a lower-resolution telescope.
The superior detail and clarity achieved through higher-resolution telescopes have significantly contributed to our understanding of the universe. High-resolution telescopes have been instrumental in various groundbreaking discoveries, allowing researchers to study objects and phenomena with an unprecedented level of precision and insight. Therefore, a higher resolution generally translates into better observational capabilities, enhancing both professional astronomical research and amateur stargazing.
Is Telescope Resolving Power the Same as Magnification?
No, the resolving power of a telescope is not the same as its magnification. However, these functions are interconnected, as increasing magnification without corresponding resolving power leads to a blurrier image. Magnification pertains to the telescope’s capability to amplify the apparent size of an observed object. Higher magnification allows observers to see more details of distant objects, but it also narrows the field of view and reduces the brightness of the image. Essentially, magnification enhances the ability to study small or distant objects by providing a closer and more detailed view.
While the magnification of a telescope increases the size of an object, it does not inherently augment the detail or clarity of the image. Resolving power denotes the telescope’s ability to differentiate between two closely situated objects, thus contributing to the sharpness and detail of the image. Therefore, while both aspects are critical in the realm of astronomical observation, they serve distinct purposes and are not the same.
Is Telescope Resolution the Same as Aperture?
No, the telescope’s resolution is not the same as its aperture, even though the two are intrinsically linked. A telescope aperture refers to the diameter of its primary optical component, which is either a mirror or a lens. The size of the aperture is crucial because it determines how much light the telescope can gather. A larger aperture is capable of collecting more light, which results in brighter and sharper images.
The resolution of a telescope, on the other hand, is a measure of its ability to distinguish fine details or closely spaced celestial objects. A larger aperture typically enables finer details to be resolved and hence a higher resolution. Though the resolution is directly influenced by the aperture, they are not identical functions.
The resolution depends not only on the size of the aperture but also on other factors such as the quality of the telescope’s optics and the prevailing atmospheric conditions. For instance, even a telescope with a large aperture will not provide a high resolution if the optics are of poor quality or if the atmospheric conditions introduce too much turbulence or distortion. Poor optics describe an aperture that is not aligned, polished or coated properly. Small flaws, such as scratches, also play an influential role in optical quality.
How Does Resolution Affect the Function of a Telescope?
The resolution of a telescope plays a pivotal role in how a telescope works, including their operational efficiency and observational capabilities. A superior resolution not only enables the telescope to discern minuscule and intricate details but also elevates the overall viewing experience. This attribute becomes particularly vital when observing celestial phenomena of complex nature, such as the intricate rings of Saturn or the vivid bands of Jupiter. These detailed observations are made possible by the higher resolution of the telescope, which allows the viewer to appreciate the intricacies of such phenomena.
Moreover, higher resolution proves invaluable when observing astronomical pairings like double stars. These are scenarios where two stars appear exceptionally close to each other from our perspective on Earth. A telescope equipped with a lower resolution will struggle to differentiate these closely positioned stars as distinct entities. Consequently, the observational experience will be compromised, with the observer missing out on the fascinating dynamics of such stellar pairings.
The resolution of a telescope fundamentally influences its observational capabilities, thereby dictating the level of detail and clarity it delivers. A comprehensive understanding of how to calculate and understand a telescope’s resolution will profoundly augment the observing experience. It empowers astronomers and enthusiasts alike to fully utilize the telescope’s potential, offering a more vivid and detailed window into the cosmos.
